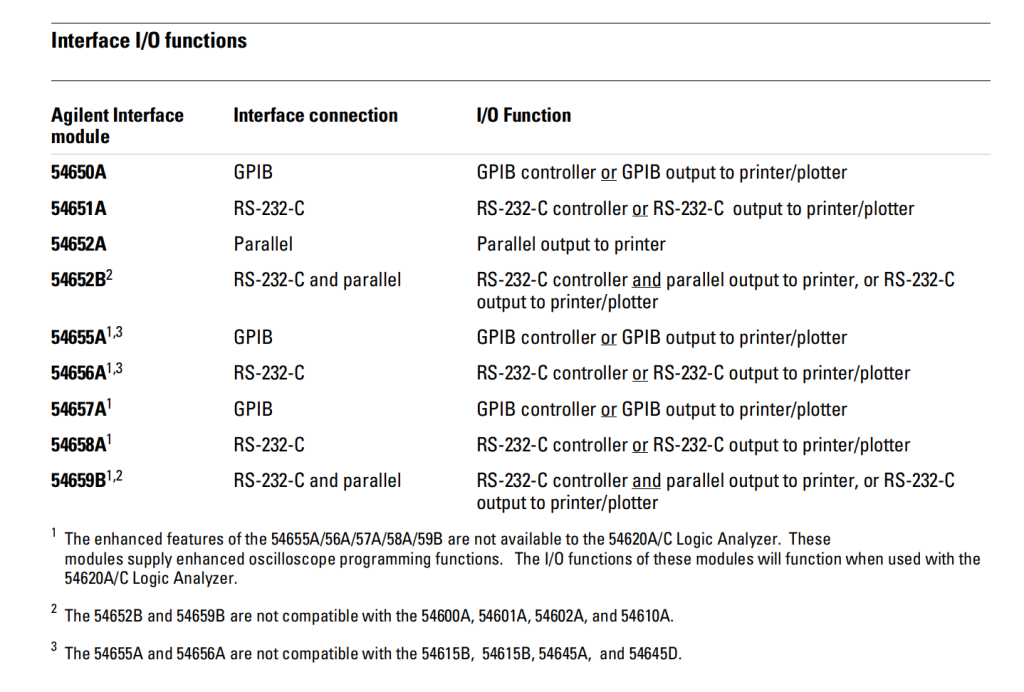
The modules are categorized into these characteristics:
- Plain (oldest, compatible with all): 54650A (GPIB), 54651A (Serial), 54652A (Parallel Printer)
- Test Automation (TAM) License/Memory: 54655A (GPIB), 54656A (Serial + 5 output lines)
- FFT/Time & Math License/Memory: 54657A (GPIB), 54659B (Serial+Parallel)
- Serial + Parallel: 54652B (no FFT), 54659B (with FFT)
The matching oscilloscopes/logic analyzers are sorted into 3 main sub-generations:
- Too Old (Cannot understand Serial+Parallel): 5460XA, 54610A
- Everything in between: 5460XB, 54610B, 54620X
- Too New (Cannot understand TAM): 54615/6B (I suspect C too), 54645A/D
Logic Analyzers (54620A/C) is considered “Everything in between” and it gleefully disregards the Test Automation/FFT features as they are only relevant to analog signals.
Only FFT modules have a RTC to keep time. TAM modules are too primative to have this.
The “Too Old” scopes have newer firmware available that handles FFT (which you need to upgrade by a chip swap if the firmware is too old), but they still don’t understand multiplexing serial & parallel lines they are stuck with 54657A.
54657A covers the broadest range of oscilloscopes (everything)
If you want the FFT and serial port together. There’s only one choice which is 54659B and you have to avold the “Too Old” oscilloscopes
It’s hard to keep track of this compatibility matrix below. That’s why this blog post explained the reasoning by categories above. It really boils down to what features that are too new (multiplexing serial+parallel port) for an old firmware and what features (TAM) the newest firmware dropped support for.
![]()